Batch 2 - Class 57 - Lets Play Dominoes

• (Moscow - 197) A complete set of dominos is as shown below. If you place all the 28 tiles in a continuous chain (adjacent ends of the tiles must match) so that 5 dots are at one end, how many dots will be at the other end?

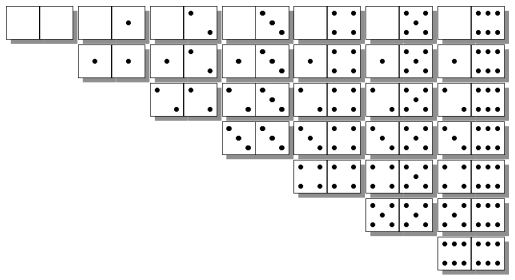
• Answer: 5 - each value appears 8 times, so inside every value must be matched. Hence, if 5 is at one end, it must be 5 at the other
• Trick: Hide one domino (not a pair), and ask kids to draw a chain of dominos. As they are drawing it, tell them what the end numbers will be :-)
• There are 6 people in a room. Each pair of people either mutually know each other or not. Prove that there are at least three people, who either all know each other, or none of whom know each other.

  Answer: Lets connect two people by a red line if they know each other and blue line otherwise. Consider any person A. A has either 3 or more red lines going out, or 3 or more blue. Without loss of generality, lets say A has 3 red lines connecting to C, D, F (any three people). If C, D, F triangle has any red lines in it (say C-D), then A, C, D forms a red triangle, hence solved. If C, D, F has no red lines, then it is a blue triangle, hence solved. Same logic applies to 3 blue lines going out of A.

• Explain the game of dominoes to kids. Double Six set

  How many pieces must the double-six set have? Lay them out in pattern above to illustrate.How many combinations of two-dice-throws exist? Why is number of dominoes different?How many pieces must a double-nine have?Blocking Game and variations
• Probability with dominoes

  Calculate probability of different sums of pips on dominoesDo you remember the probability of sums on a dice? For example, what are chances of 7-roll on two dice?
• Tiling with dominoes

  Give kids a 5x6 square grid, and 15 2x1 dominoes. Ask them to tile the grid with dominoesPossible/ Not Possible?Do they have the same answer?If not, how many answers exist?What if it is a 6x6 grid and 18 dominoes?Answer: Trivial case of all horizontal or all vertical dominoes
• Fun with domino topple

  Try out a small sequenceWorld record 275,000 dominoes in a spiral https://www.youtube.com/watch?v=1QtdPfz_faMGuiness record 543,210 dominoes https://www.youtube.com/watch?v=16eF8IQzgP8Domino Magnification - a domino can knock over a domino 1.5x its size https://www.youtube.com/watch?v=y97rBdSYbkg 
• Brick Wall - In how many ways can you tile a brick wall of 2xn dimension using 2x1 dominos?

  Try for small numbersCan you generalizeHint: Think about the first element on the wall - how many choices exist?See if some kids can get to fibonacci
• Domino Art work

  Some simple patterns here http://www.mathematische-basteleien.de/dominos.htm 

  

  You can build your own domino artwork at http://www.dominoartwork.com/downloads.html

•  (Arvind Gupta 19) Use eighteen of the dominoes from a standard double-six set to form a 6 x 6 square in such a way that the number of dots in each of the six rows and columns and the two main diagonals is the same. 

• Can you tile a 6x6 grid with 2x1 dominos, so that there are no fault lines (i.e. any horizontal or vertical line has at least one dominos going across it?)

  Answer: Can't be done - each of 10 lines in 6x6 grid divides the region into two even areas, so at least two dominoes must cross it. So there must be at least 20 dominoes, but there are only 18